Introduction
Soil, the most precious resources to humans and the earth’s skin comprised of countless species, is a dynamic and complex ecosystem. Environmental functions of soil which is affected by soil erosion is very real and severe issues in terms of soil quality at times. Larson and Pierce (1994) define soil quality as the capacity of a soil to function, both within its ecosystem boundaries and with the environment external to that ecosystem. On the impact of erosion at the surface soil horizon and landscape scales, the primary focus is on the concept into the soil erosion-soil quality relationship. The aspect of assessing the seriousness of erosion involves the impacts it has both in-field and off-site, and what the economic costs of these effects are (Boardman and Poesen, 2006). Increased soil loss rates due to wind constitute an on-site challenge that decreases the ability of soils to sustain vegetation and livestock (Goossens, 2003). At the same time, it also causes off-farm impacts related to the spread of dust, herbicides and pesticides (Riksen and de Graaff, 2001).
Wind erosion, the primary cause of natural soil degradation process exacerbated by human activities, damages the soil by physically removing clays, silts, and organic matter across a field. Wind erosion is initiated with the wind speed exceeding the saltation threshold velocity for a given soil and biomass condition can degrade soil, air, and water resources, and damages soil structure and water holding capacity (Lyles and Woodruff, 1960; Armbrust, 1984). The duration and severity of an erosion event after initiation of soil erosion by wind depends on the wind speed distribution and the evolution of the surface condition (Hagen et al., 1988; Hagen and Foster, 1989).
The results of recent studies indicate that few details are known about the magnitude of wind erosion. Intrinsic soil properties such as texture, mineralogy, and organic matter content moisture determine availability of loose erodible material, while the potentially erodible area of a landscape is controlled by the density and distribution of vegetation cover and other roughness elements that shelter the soil surface (Smalley, 1970; Breuninger et al., 1989; Zobeck, 1991). Soil erodibility which is the most important tool for estimation the erosion is influenced by spatial scales due to the change over time and the combined factors of intrinsic soil properties that vary through space and time, the state of a soil surface within a continuum, extending from minimum to maximum erodibility (Greeves et al., 2000, 2007). However, the current erosion research does not provide answers about the “where” and “when” of wind erosion especially in nonagricultural lands (Borrelli et al., 2015).
Wind erosion models are designed to simulate on-site and in some cases off-site consequences of soil loss for given land conditions (Tatarko et al., 2016). Therefore, further investigation in the development of wind erosion models is needed to more accurately predict and estimate soil loss by wind erosion depending on through space and time. The objectives of this study are to evaluate the applicability of the wind erosion models of wind erosion equation (WEQ), revised wind erosion equation (RWEQ), and wind erosion prediction system (WEPS) in terms of spatial and temporal patterns by investigating and ascertaining applications related to some weaknesses and areas
Concepts and models of soil wind erosion
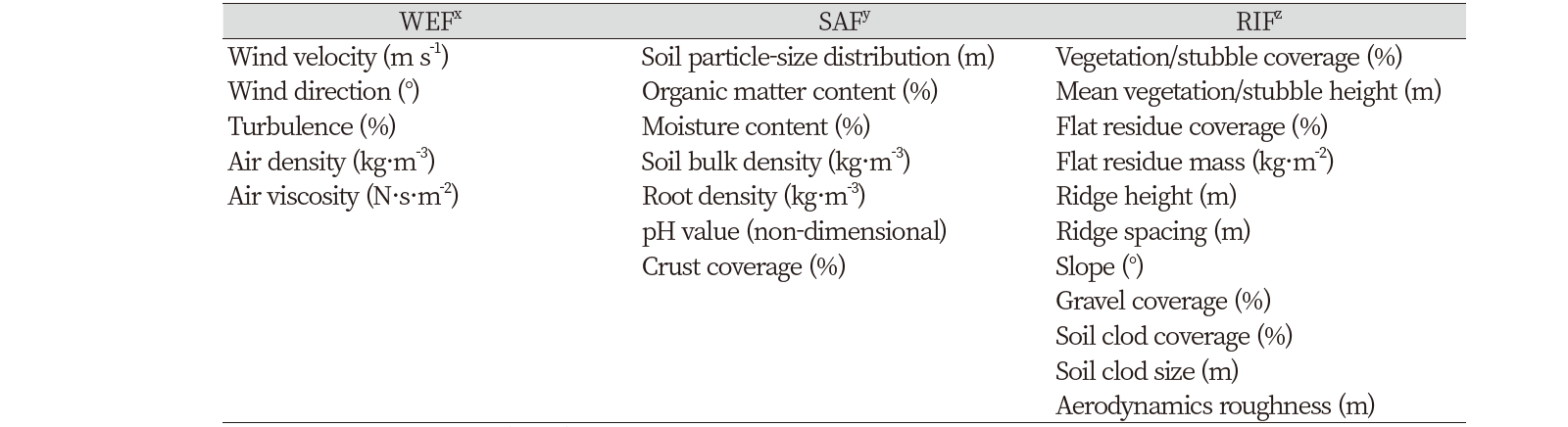
Wind erosion which is one of the links including three main links, namely erosion, transportation, and deposition is a complex geomorphic process governed by a large number of variables (Liu, 1995; Zobeck and Van Pelt, 2006). Soil loss by wind is a concept with a time, length, and spatial scale (Woodruff and Siddoway, 1965). The most commonly used index of soil loss by wind is the soil wind erosion modulus, i.e., the amount of soil loss by wind per unit time and unit area, resulting in that soil loss by wind represents the temporal and spatial average of the “Point” flux (Zou et al., 2014). For factors classified into three categories (air, ground, and soil) in the process of soil wind erosion by Chepil (1945a, 1945b, 1945c), Zou et al. (2013) revised these factors into three categories, namely wind erosivity factors (WEF), soil antierodibility factors (SAF), and roughness interference factors (RIF), corresponding to wind driving force, soil counteraction force, and roughness interference force (Table 1).
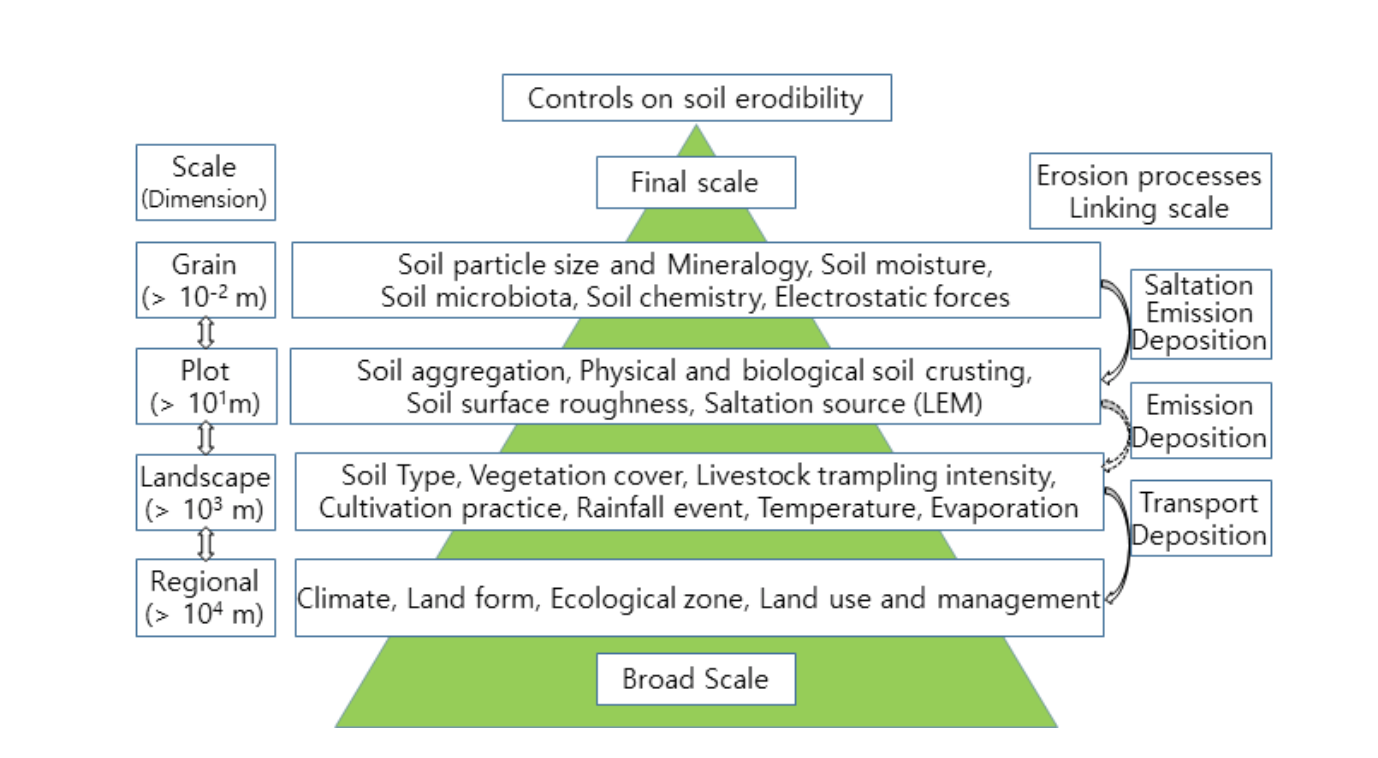
Fig. 1. Diagram illustrating controls on soil erodibility at different spatial scales, including within and between the soil grain (< 102 m), plot (101 m), landscape (103 m) and regional (104 m) scales. Arrows down the right-hand side of the figure show the erosion processes that functionally connect the scale domains (Source: Webb and Strong, 2011).
The susceptibility of a land surface to wind erosion is highly sensitive to changes in the soil erodibility (Borrelli et al., 2016) which is controlled by intrinsic properties of soils, and the combined influence of temporal soil properties of moisture, aggregation, surface crusting and the availability of loose erodible material (Zobeck, 1991). Cover and landscape roughness although relations among variables are extremely complex. The erodibility of soils within the erodible area of a landscape vary across spatial scales, and is influenced by: (1) environmental conditions of soil type, landform, climate and ecological zone; and (2) land use and land management practices (Okin et al., 2006; Okin, 2008). Soil erodibility is therefore represented by processes operating at the smallest spatial scale in wind erosion models (Fig. 1) that tend not to accommodate temporal variations in soil erodibility controls at the plot, landscape or regional scales (Webb and Strong, 2011).
Actual observations, field measurements and modelling assessments, however, are all extremely limited and highly unequally distributed across the field (Borrelli et al., 2015). The researches to develop the wind erosion model began with single factors in the mid-1950's and Chepil’s group released the empirical equation in 1962: E = f (A C K L V), where, A is the percentage of soil fractions greater than 0.84 mm. Factors C, K, L, and V were the same as in the present equation although they were not handled the same. The current form of the equation expressed as E = f (I K C L V) was released in 1963, where E is the estimated average annual soil loss; I is the soil erodibility index (Woodruff and Siddoway, 1965; NRCS, 1988, 2020); K is the surface (ridge) roughness factor; C characterizes climatic erosivity, specifically wind speed and surface soil moisture for any given locality. L is the field length, and V is the vegetative factor expressed in pounds per acre of a flat small-grain residue equivalent. In this equation, wind velocity at geographic locations was not addressed. The first wind erosion model WEQ (Woodruff and Siddoway, 1965) was designed to determine (i) if a particular field is adequately protected from wind erosion, and (ii) the different field conditions of cloudiness, roughness, vegetative cover, sheltering from wind barriers, or width and orientation of field required to reduce potential soil loss to a tolerable amount under different climates.
Continuous researches became available modified and advanced models to quantitatively evaluate wind induced soil erosion. Several revisions were made for specific factors in the WEQ framework due to the need for management factors impacting soil erosion of WEQ (Tatarko et al., 2013), including stubble and non-erodible aggregates (Lyles and Allison, 1975), crop type and tillage affecting the properties of soil aggregates (Armbrust et al., 1982), the soil ridge roughness factor (Lyles and Tatarko, 1987), and the soil erodibility index used in different seasons (Lyles and Tatarko, 1988) and accounting for the wind erosion direction and the preponderance of wind erosion forces (Skidmore, 1987). Then, the WEQ was modified into RWEQ to permit the estimate of soil erosion for time periods shorter than one year in the beginning of 1980s (Bondy et al., 1980; Fryrear et al., 1998a; Fryrear et al., 1998b). The models developed are RWEQ, WEPS, single-event wind erosion evaluation program (SWEEP) etc. at a field scale and wind erosion assessment model (WEAM), integrated wind erosion modeling system (IWEMS), Australian land erodibility model (AUSLEM) etc. at a regional scale. These models were mainly used on agricultural areas and a wind erosion assessment spatial model, capable of covering large territories, was not available until the year 2000 (Mandakh et al., 2016). Among these models, WEQ (Woodruff and Siddoway, 1965), RWEQ, (Fryrear et al., 1998a), and WEPS (Hagen, 1991) were the most common models used to predict wind erosion at the field scale. WEQ and RWEQ utilize the same basic inputs although the technology using these inputs is entirely different. AUSLEM which was the first spatial model developed in Australia did not account for soil moisture levels or surface roughness, two important components in empirical models although it was designed to assess climatic and terrain surface factors from satellite data (Leys et al., 2001). However, the challenge of precisely estimating wind erosion at a regional scale still remain to date.
WEQ The WEQ was the only empirical and spatial model used on large territories including agricultural areas for over 30 years (Mandakh et al., 2016) until the RWEQ was published in 1998 (Fryrear et al., 1998a). The WEQ was developed from wind tunnel experiments and limited field observations to predict long-term average annual soil losses from a field having specific characteristics although this does not allow users to identify critical periods within a rotation where wind erosion may be higher than recommended (Buschiazzo and Zobeck, 2008). The WEQ is a prediction model as a simple exponential expressing the amount of soil loss in a wind tunnel as a function of percent soil cloudiness, amount of surface residue and degree of surface roughness to determine the primary variables or factors influencing erosion of soil by wind (Woodruff and Siddoway, 1965). The equation was expressed in terms of equivalent variables as: E = f(I K C L V) where I is a soil erodibility index at a location where the climatic factor (C) is equal to 100 and accounts for the inherent soil properties including texture, organic matter, and calcium carbonate percentage. K is a soil ridge roughness factor as a measure of the effect of ridges and cloudiness made by tillage and planting implements. C includes the wind regime, rainfall, temperature and humidity, with the wind velocity for any given locality, L is the unprotected distance along the prevailing erosive wind direction across the area to be evaluated, and V is equivalent quantity of vegetative cover, indicating the relation between potential soil loss from a field and climatic variables as seen in Table 2 (Woodruff and Siddoway, 1965; Webb and Strong, 2011).
Solving this equation involves five successive steps. Steps 1, 2 and 3 can be solved by multiplying the factor values as seen in Table 2. Determining the effects of L and V (steps 4 and 5) involves more complex functional relationships However, the equation does not only account for snow cover or seasonal changes in soil erodibility, as well as the equation does not estimate erosion from single storm events. Thus, the present equation has significant limitations, it is considered as the best tool currently available for making reasonable estimates of wind erosion. According to Woodruff and Siddoway (1965), the annual amount of soil eroded from a given field is a function of the factors listed in Table 3.
Table 2. Input parameters for the wind erosion equation (weq) and the revised wind erosion equation (RWEQ) (Source: Fryrear et al., 1999).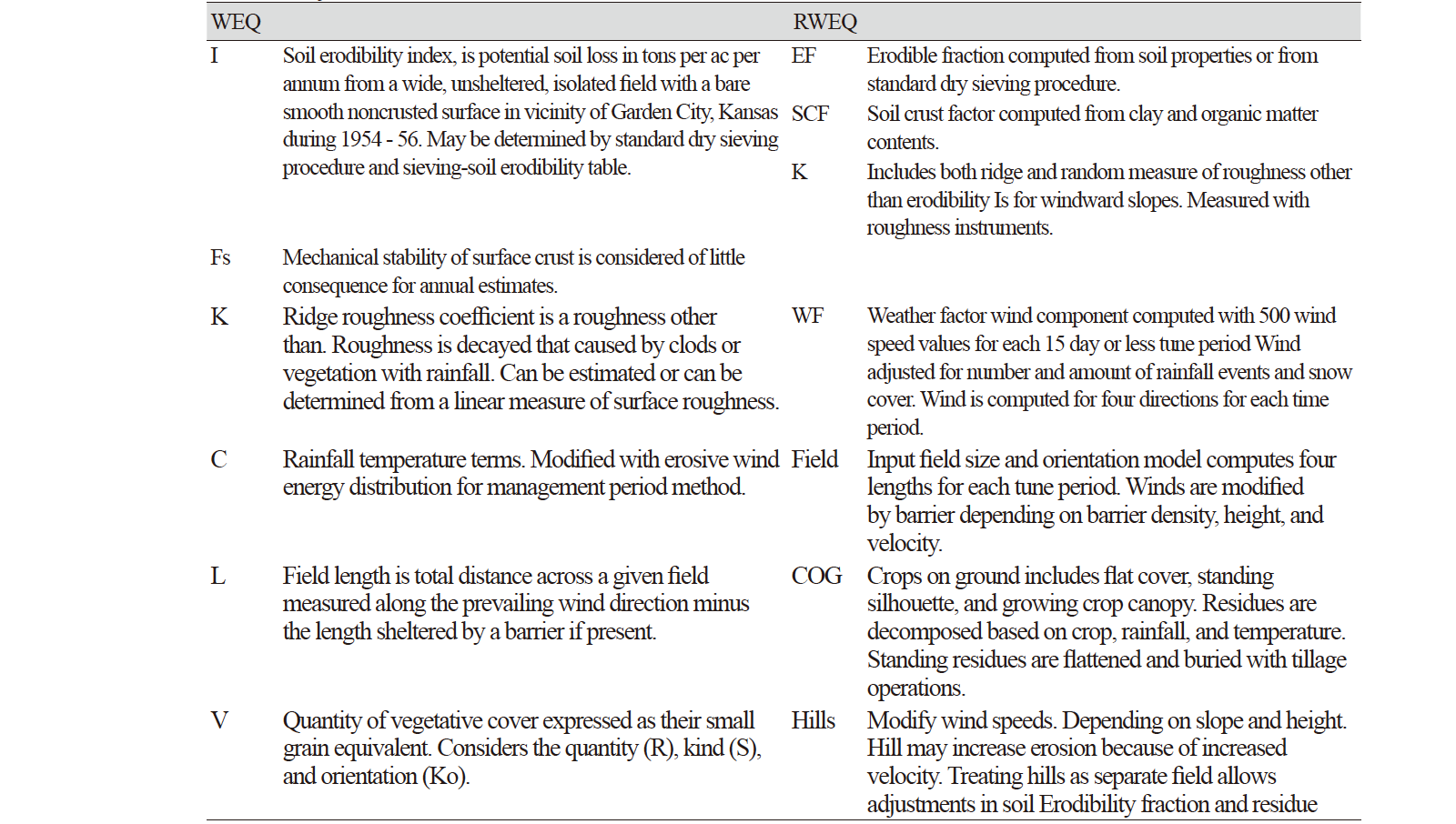
|
Table 3. Five successive steps to solve wind erosion equation (WEQ) equation and functional relationships.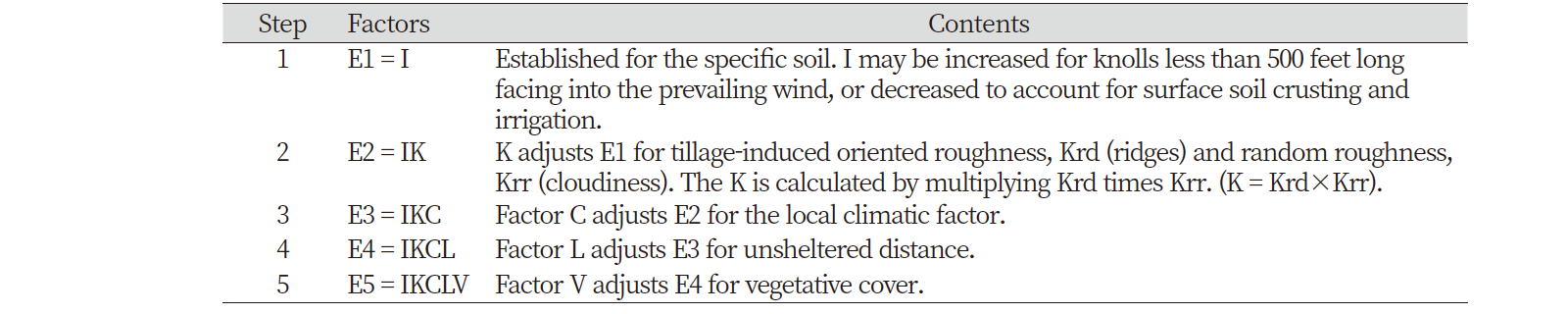
|
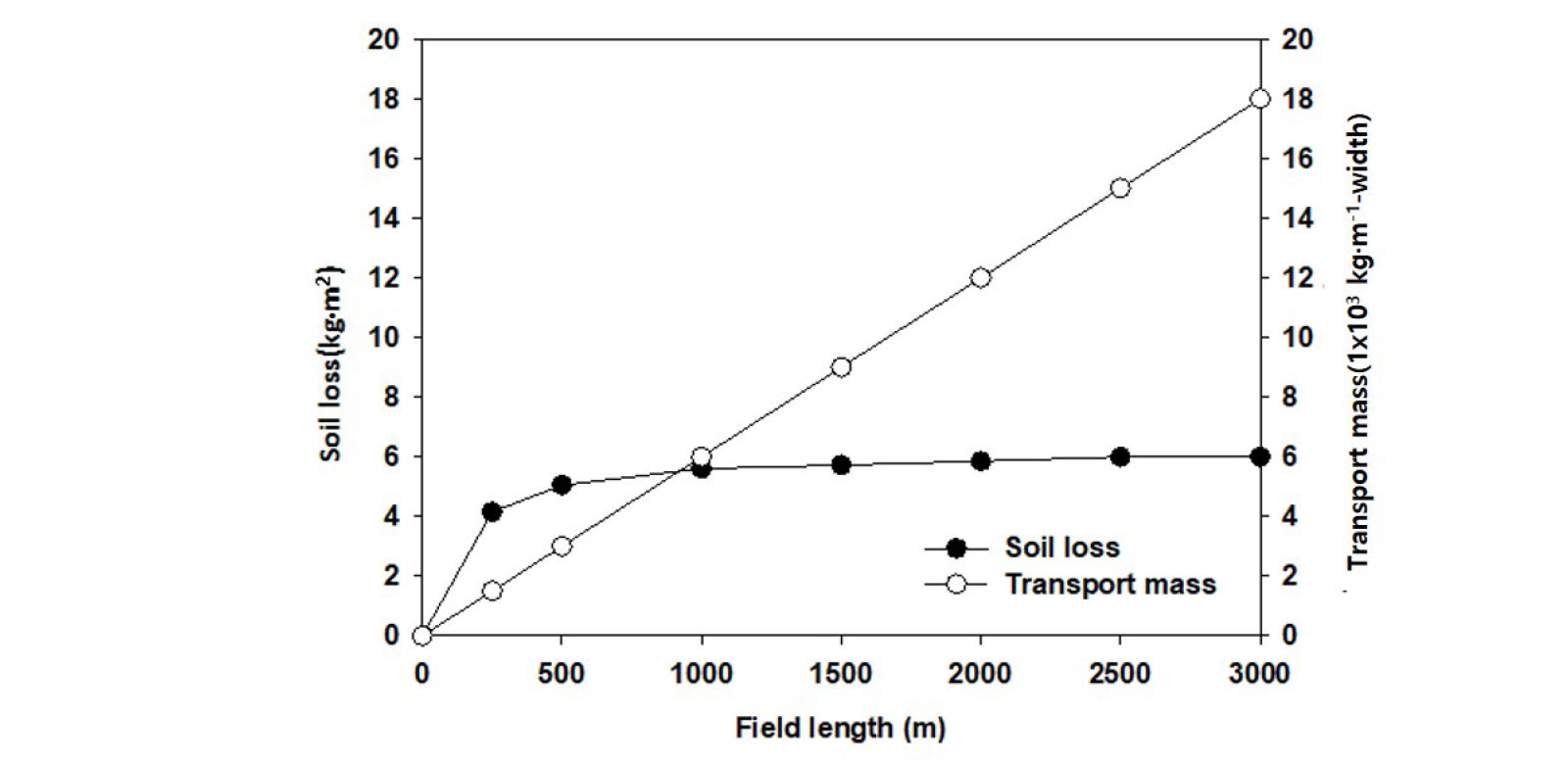
The existing validation studies of WEQ have produced inconsistent results. Several researchers found that WEQ is not accurately representing soil erosion and under- represents soil erodibility that consequently results in WEQ underestimations (Huang et al., 2017). With WEQ, the transport mass must increase without limits for the average soil erosion (Fig. 2) to remain constant for large fields. This does not agree with Bagnold's theory (1937, 1941) that the wind has a limited capacity to transport material and Chepil (1956, 1958) statement that rate of soil flow increased with distance downwind until it reached a maximum that a wind of a given velocity can carry if the field was large enough. Also, it is not possible to estimate any erosion regardless of soil texture if the climatic factor drops below 10 (Fryrear et al., 1999).
In addition, Fryrear et al. (2000) concluded that uncertainties of WEQ associated with climatic factor (C) and soil erodibility (I) were responsible not only for poor WEQ prediction but also for underestimation of wind erosion in areas with precipitation less than 800 mm. Van Pelt et al. (2004) found that the effect of climatic factor on erosion was not expressed strongly enough in the WEQ model due to a small I-factor value for WEQ’s underestimation (e.g., 53.3% of the observed estimated erosion), but the differences in observed estimated erosion between the two years was much greater than in the predicted erosion (Buschiazzo and Zobeck, 2008). A detailed sensitivity analysis on WEQ is needed to identify the primary factors affecting its performance. In terms of the shortcomings of WEQ, there were several revisions to specific factors in the WEQ framework as observed above. These revisions improve estimates of erosion, allows more flexibility in inputs, and enhances output. Huang et al., (2017) reported that the WEQ can be used as a reliable prediction model for long-term predictions of wind erosion in the semi-arid pampas, even when it runs with the limited climatic data.
RWEQ The RWEQ model is based on WEQ and contains both empirical and process-based components with the ability of describing physical wind erosion processes by combining field data sets with a computer model for the prediction. The RWEQ is most applicable for erosion from the field and provides information on erosion rate effects within the field although this model is not completely a physically-based model (Blanco-Canqui and Lal, 2008; Fryrear et al., 1999). The RWEQ is a combination of empirical and process modeling to estimate soil eroded and transported by wind between the soil surface and a height of two meters in a field where wind limits total erosion (Fryrear et al., 1998a; Fryrear et al., 2000). The relationship between the mass of transport, field length, and soil loss is basic of this definition. It makes annual or period estimates of wind erosion based on a single event wind erosion model that includes factors for wind and rainfall, soil roughness, the erodible fraction of soil, crusting, and surface residues (Van Pelt et al., 2004). However, it is not applicable for those problems were suspended, fine sediment above two meters is the concern.
The RWEQ uses mathematical equations and associated parameter values to compute estimates of soil eroded and transported by wind for that portion of sediment that moves between the soil surfaces up to a height of two meters (Gregory and Borrelli, 1986; Stout, 1990; Hagen and Armbrust, 1994; Fryrear et al., 1998a). The amount of mass transport varies with soil texture, soil surface, field length, and climatic conditions (Fryrear and Saleh, 1996; Stout and Zobeck, 1996). The mass of eroded soil material being transported by wind depends on the magnitude and duration of the wind speed, soil erodibility, orientation and quantity of crop residues, and the type, timing and number of tillage operations. These equations assume that the horizontal flux is proportional to the difference between the maximum transport and the actual transport at a point within the field. As shown in equation below, average soil loss is defined as mass transport at field length x or Q(x) divided by distance x. To calculate Qmax and s, the coefficients for weather, soils, crops, and tillage are needed.
Soil loss = 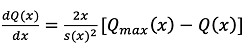 (1)
(1)
Where, Q(x) = mass transport at downwind distance x, (kg·m-1-width), Qmax(x) is maximum transport (kg·m-1-width), Q(x) is amount of transport (kg·m-1-width), S(x) is surface retention coefficient, x is distance from the upwind edge of the field (meters).
A number of studies have found good agreement between the yields predicted by RWEQ and the field measures (Buschiazzo and Zobeck, 2008; Youssef et al., 2012). The significant relationship between the observed and predicted transport capacity and soil loss (Zobeck and Bilbro, 2001), as well as the limited need for input data compared with mechanistic wind erosion models like the WESP (Hagen, 2004), makes RWEQ a suitable tool for a large scale prediction of the wind erosion potential (Zobeck and Bilbro, 2001; Youssef et al., 2012; Guo et al., 2013). RWEQ utilizes monthly weather data, soil and field data, and management inputs. The management inputs include cropping systems, tillage and operation dates, wind barrier descriptions, and, where applicable, irrigation information. With RWEQ, estimates of erosion will be much closer to measured values for any cropping system or climatic region of the country than estimates of erosion with WEQ (Fryrear et al., 2000).
WEPS Because of their empirical and complex nature, WEQ and RWEQ were not easily adapted to conditions or climates different from the US central Great Plains (Hagen, 1991; Jarraha et al., 2020). WEPS was developed to replace the predominately empirical WEQ as a field-scale prediction tool and physically-based daily simulation model and is designed to be and aid in: 1) soil conservation planning, 2) environmental assessment and planning, and 3) determining off-site impacts of wind erosion. The WEPS represents a potentially significant improvement in comparison to previous wind-blown dust modeling algorithms that employed only simple physics and relied on empirical formulations (Nickovic et al., 2001; Hagen, 2004; Shaw et al., 2008). The WEPS is useful tools for non-agricultural lands where typical agricultural practices are not employed (Tatarko et al., 2016). WEPS is capable of outputting erosion loss and surface conditions on a relatively fine temporal scale (e.g., hourly). It is a process-based, continuous, daily, time-step model that simulates not only the basic wind erosion processes but also the processes that modify a soil's susceptibility to wind erosion (Hagen et al., 1996; Tatarko and Wagner, 2007).
WEPS contains subroutines in a separate file as a modular design to allow individual components of the WEPS submodels (Table 4) to be easily maintained, modified, or replaced if necessary (Hagen et al., 1996). Submodels that simulate the surface state include: hydrology, soil, management, crop growth, residue decomposition. These submodels provide and update parameters dynamically and supply the erosion submodel information needed to calculate soil loss (Jarraha et al., 2020). The WEPS simulates weather, field surface conditions, and wind erosion model by employing up to some tens of parameters to predict long-term soil productivity by soil loss, determines physical damage to crops by soil loss, calculate soil movement, estimate plant damage, and predict Pm-10 emissions when wind speeds exceed the erosion threshold (Wagner, 2013). It also can provide the user with spatial information regarding soil flux, deposition, and loss from specific regions of a field over time (Wagner, 1996, 2011; Tatarko and Wagner, 2007). WEPS' seven submodels, each based on the fundamental processes which occur in the field, are used to predict and give estimates for wind erosion. They consist of: 1. Weather (rainfall parameters, temperature, solar radiation and wind), 2. Crop growth (cropland, rangeland, forestland), 3. Decomposition (rills, channels, and impoundments), 4. Hydrology (irrigation, infiltration, depression storage, runoff), 5. Soil (types and properties), 6. Erosion (in terrill, rill, channel), and 7. Tillage.
WEPS requires only four inputs to make a simulation: (1) the field size, shape and orientation; (2) the soil type; (3) the management/crop rotation practice applied; and (4) the climate experienced at the site. With these inputs, WEPS can make a multi-year simulation run, which will reflect the seasonal and year-to-year variability of the climate. WEPS can also provide estimates of the long-term erosion rate and can allow the user to identify when the site is most susceptible to erosion during the crop/rotation cycle (Wagner, 2011). He also reported that there were good agreements (i.e., coefficients of determination ranging from 0.87 to 0.98) between measured and WEPS-simulated erosion in a number of studies (Buschiazzo and Zobeck, 2008; Liu et al., 2014). However, the model estimated zero erosion when small amounts of erosion occurred when test site conditions are not uniform and have small inclusions with higher erodibility than the average conditions that are assumed in the model inputs (Hagen, 2004). In several studies WEPS was found to under-predict or not predict some relatively small erosion events on soils covered with plants, residues, or had a high surface roughness (Funk et al., 2004; Feng and Sharratt, 2007; Feng and Sharratt, 2009; Pi et al., 2014). van Donk and Skidmore (2001) also suggested that WEPS needs a provision to account for standing biomass that is not uniformly spaced, such as wheat plants in the field. Albedo is a good indicator of soil wetness right at the soil surface. Additional research is needed to use albedo for quantifying soil surface water content.
Comparison of applicability for WEQ, RWEQ, and WEPS
For the models investigated, there are many possible explanations for the variation in the differences between the model predictions and the observed estimates. The WEQ is an empirical and spatial prediction model based on limited field observations while the RWEQ is a combination of empirical and process model to describe the wind erosion process and the wind erosion potential for areas with annual rainfall from 191 to 915 mm and makes annual or period estimates of wind erosion based on a single event wind erosion model. WEQ and RWEQ utilize the same basic inputs although the technology using the inputs is entirely different. WEPS is a physically-based field-scale and multiple process-based, daily-time step prediction model (e.g., hydrology, plant growth and decomposition, long-term erosion control strategies) to predict wind erosion soil loss as affected by site-specific climate, soil type, and land management (Table 5).
Table 5. Structure of the wind erosion prediction system (WEPS) submodels (Source: Modified from Jarraha
et al., 2020).
|
The validation studies showed that no statistical correlation between erosion estimates from RWEQ and WEQ for numerous management systems and climatic regions. WEQ only predicts soil loss for a single, uniform, isolated field. WEQ, therefore, does not only accurately represent soil erosion and under-represents soil erodibility that consequently results in WEQ underestimations but also it is not possible to estimate any erosion regardless of soil texture if the climatic factor drops below 10. WEQ does not specify the height while transport mass by RWEQ is the mass of soil being transported by wind in a band of unit width from the soil surface to a specific height of 2 meters. WEQ weather is from the closest location, but soil erodibility I was based on measured soil properties at the site. The correlation between measured erosion and estimated erosion with C factor and rainfall of WEQ was not significant, resulting in that the WEQ should be used with variable C factors and rainfall in order to assess different climatic conditions. RWEQ erosion estimates reflects the importance of leaving residues stand. The RWEQ computes residue decomposition and decays soil roughness to reflect current field conditions and simplifies erosion computations, based on soil and climatic conditions. The output of WEQ is the average soil erosion that could occur from a given field length. The output of WEPS which consists weather, crop growth, decomposition, hydrology, soil, erosion, and tillage shows erosion loss and surface conditions on an hourly scale. In several other cases, the WEPS estimates zero erosion when site conditions are not uniform and have small inclusions with higher erodibility than the assumed average conditions in the model inputs. Estimation of soil loss by WEPS and RWEQ for single event in a bare soil condition showed that both models tended to underestimate the observed wind erosion measurements due to the use of different equations for calculating the horizontal mass flux and sensitivity to variations in soil surface crusting.
Summary
Wind erosion models are generally designed to simulate on-site and in some cases off-site consequences of soil loss for given land and climatic conditions. There are many variations in the differences of predictions and the observed estimates among the soil wind erosion models. By analyzing the various factors related to the soil wind erosion, we can assume that WEQ can be used to predict only long-term, average soil loss for a single, uniform, isolated field, RWEQ is suitable for a large scale prediction of the wind erosion potential by estimating closer values for any cropping system or climatic region where suspended, fine sediment are less than two meters, and WEPS calculates soil loss on a daily basis provides capability to handle nonuniform areas on a relatively fine temporal scale (e.g., hourly). The largest contrast between WEPS and WEQ is that WEPS simulates a wide range of processes to describe field surface conditions and wind erosion, whereas WEQ depends on users to input correct estimates of the field surface conditions. Therefore, the RWEQ and the WEPS are suitable to be more realistic estimates of erosion because these models can use easily measured local soil and climatic variables as inputs including for specific soil, crop, and management actions. Also, the significant relationship between the observed and predicted transport capacity and soil loss makes both RWEQ and WEPS to look inside the simulation region to obtain predictions for specific areas of interest. Finally, this research found that the suitable temporal and spatial settings for assessing wind erosion rate is one of the major elements to decrease the uncertainty of the soil loss and show better model accuracy for better nationwide predictions.