Introduction
Radishes (Raphanus raphanistrum subsp. sativus) are a vegetable widely grown throughout the world, particularly in South Asian countries like Korea, Japan, and China. The annual radish production in Korea was 0.365 and 0.467 million tons in 2020 and 2021, respectively, which was a 9.8% increase in total production (KOSTAT, 2021). Radishes are cultivated in open fields as an annual or biannual crop, and are a good source of vitamins, minerals, and other nutrients (Chowdhury et al., 2021). In many countries, radish cultivation is declining due to labor-intensive and time-consuming cultivation, aged labor with high labor expenses, and limited automation. Field operations such as cultivation and harvesting demand a significant number of human resources. The most labor-intensive activities involve manually picking, gathering, and packing radishes (Jang et al., 2021; Reza et al., 2021; Swe et al., 2021a). Due to significant economic and industrial expansion in the Republic of Korea, agricultural mechanization has been implemented in an effort to alleviate the scarcity of agricultural employees. The mechanization rate of all major upland crops reaches an average of 50%, but the rate of sowing, transplantation, and harvest stages (i.e., where labor is most concentrated) is only 6.7, 7.2, and 25.8%, respectively, and in the case of the harvesting stage, the mechanization rate may decrease by up to 8.9%, which is the lowest rate among all upland crops (Yoo and Hwang, 2018). Therefore, radish collectors are in desperate need of mechanization through the use of agricultural machinery to reduce time and reliance on human labor throughout various field operations.
Continuous attempts to improve agricultural machinery have inspired numerous studies, and it is necessary to pay close attention to all of the factors involved in this issue. Research has been carried out in order to produce a range of harvesters for upland crops, with the goal of reducing crop management problems while simultaneously maximizing crop yield. Yaegashi et al. (2001) designed a radish harvester after analyzing its performance in various field conditions, and Guolong et al. (2016) developed a radish harvester based on a digging shovel model developed by theoretical analysis to achieve the finished harvester. Different types of radish harvesters are being studied for future improvement; researchers want to learn more about the ideal configurations for the conveyor and depth controlling plate, as well as the digging conveyor, primary and secondary cutting blade, and gearbox through the use of field tests (Jung et al., 2018). Particularly, research on the self-propelled radish harvester was not conducted in Korea, but was developed as a tractor-attached type. In light of how many radishes are grown in Korea and how the land is used, it is important to continue developing the self-propelled radish harvester (Kook et al., 2021).
Fatigue life is described as how long an object or substance will last before it completely breaks down due to stress, and is usually calculated as the amount of stress cycles that an entity or material may endure before the damage is done. Fatigue life relies on the running characteristics, model parameters, substance features, and load conditions (Köksal et al., 2013; Swe et al., 2021b). Researchers were introduced to the load spectrum from which the failure life of a machine substance could be estimated. For the purpose of counting the cycles of the observed load loops, the rain flow cycle-counting technique was considered with the sequence effect (Jung et al., 2000).
Due to repetitive loading, components of a tractor-mounted radish collector will propagate a fatigue crack in a high-stress zone until ultimate breakage occurs (Milan et al., 2020; Islam et al., 2021). Upland farm machines such as the onion harvester (Shin et al., 2019) have been improved and utilized in trials based on normal working speed during operation. Strain was measured from 8 different points of a tractor-linked onion harvester and converted into stress in order to achieve the optimum safety factor. Gujar and Bhaskar (2013) presented the fatigue life prediction based on finite element analysis (FEA). The motor shaft is a rotating device, and the stress on a particular spot of the motor shaft changes as the shaft rotates, creating fatigue in the shaft.
Agricultural machinery, especially tractors and various tractor-mounted components, frequently uses welding joints. The material is heated and then cooled throughout the welding process. Physical and chemical differences result in the formation of holes and hollows due to the fundamental components being melted and merged with the filled product. The cross section of the welded joints without a base produce a large range of stress, which leads to fatigue damage of the joint (Eggert et al., 2012).
The tangential tensile stress zone on circular cutting blades used in agricultural machines can increase the circular blade's longevity (Li et al., 2015). To ensure dependability, the narrow, circular saw blade requires a higher tangential tensile stress and a lower radial compressive tensile stress. The implications of strain on the blades’ dynamic dependability warrant investigation (Zhang et al., 2014; Gospodaric et al., 2015). The radish collectors’ operational parameters were focused on annual working hours, working velocity, stress, load distribution, and fatigue life.
A tractor-mounted radish collector is under development, and the major components of the collector require evaluation in terms of mechanical properties and fatigue life in order to effectively run the collector throughout a suitable service life-range. The analysis of the major functioning components of the harvesters considered as a whole is still in its early stages and requires more research. Therefore, the objectives of this study are to analyze the stress and fatigue of motor shafts for the stem-cutting conveyor belt, transfer conveyor belt, winch motor shaft, transportation joint, and circular cutting blade of radish collectors, and to forecast the expected service life, design, and dimension of the materials.
Materials and Methods
Structure and working procedure of the radish collector under development
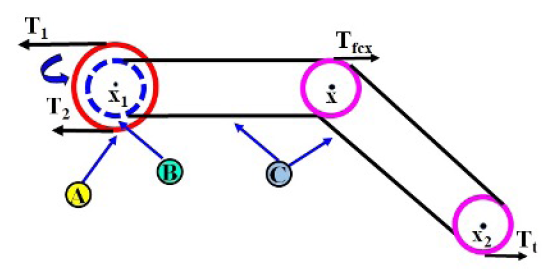
Fig. 1 shows the 3D model of the radish collector which was attached to the tractor during the field test, and Fig. 2 illustrates the tension forces on the transfer conveyor of the collector. The collector is composed of 8 key components: the stem-cutting conveyor, transfer conveyor, three hydraulic motors for conveyor belts and stem-cutting, direct current (DC) winch motor, and two furrow guides. Traditionally, farmers harvested radishes by placing them on the stem-cutting conveyor. The radishes were then carried through the transfer conveyor, where polypropylene bags were positioned at the end to collect them, after which the full bags were moved to the desired location. The winch motor was used to fold and unfold the stem-cutting conveyor before and after the operation. The mass of the prototype collector was 460 kg, and the dimensions were 4.21 m × 1.20 m × 2 m (L × W × H), respectively. The average mass and length of the radishes were between the range of 0.14 to 2.27 kg and 0.15 to 0.61 m, respectively.
Procedures for stress behavior and severity level of the components
An experimental study was conducted to determine the shaft torque of belt motors used in the stem-cutting and transferring conveyors. Considering field condition, the stress behavior of two motor shafts for two conveyors and a winch, transportation joint, and stem-cutting blade were determined with and without load conditions. For the appropriate shear stress with distribution of fatigue life cycles, the Smith-Watson-Topper mean stress equation and the Rain-flow-counting method were evaluated. Miner's rule was used to determine the severity of the operation by calculating the ratio of the total damage fraction.
Experimental torque measurement of hydraulic motor shafts
Power is transmitted by a shaft, which is a rotating part with a circular cross-section. Rotor system failures are frequently caused by a fatigue fracture in a hydraulic motor's rotating shaft. For long-term functioning of the machine components, the significant results will guide the protection model and anticipate the fatigue lifecycles (Niu and Yang, 2018).
This study was supervised by the Department of Agricultural Machinery Engineering in the College of Agriculture and Life Sciences at Chungnam National University, and the Daejeon and Rural Development Administration (RDA) in South Korea. The experiment was carried out on August 7th, 2019, and the experimental site was located at Pyeongchang-gun, Gangwon-do, South Korea. The experiment was performed on a test area of 800 m2, where radishes (Raphanus sativus) were grown. At the time of harvest, the field appeared to be in good condition.
Two torque sensors (Futek TRS-605, California, USA) were connected to two 12 kW motors (MP250, Kazanluk, Bulgaria) via the LabVIEW (National Instruments Corp., Austin, Texas, USA) data acquisition (DAQ) system, which included programs for rotational speeds of 42, 87, and 112 rpm, as well as for loaded and unloaded conditions. The experiment was carried out using tractor forward speeds of 1.36, 1.55, and 1.73 km·h-1 and an engine power of 52 kW. Each trial was carried out three times under identical conditions.
Analysis of stress behavior of conveyor motor shafts
American Society for Testing and Materials (ASTM) standard for SCM420H carbon steel was used to replicate the S-N correction data of the motor shafts for various operating and tractor ground speeds in both loaded and unloaded conditions by equation (1) (Kim et al., 2013).
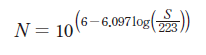 (1)
(1)
Where, N is the number of cycles and S is the shear stress (MPa).
The tensile and fatigue strengths of the substance were assumed to be 1,817 MPa and 700 MPa, respectively (Lee et al., 2018). The effects of the radish collector operations on the fatigue life of the motor shafts were evaluated using the partial damage theory, and were compared in terms of severity level on the material’s fatigue strength. The equivalent torque of the load spectrum was transformed into shear stress using equation (2) (Petracconi et al., 2010).
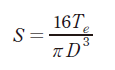 (2)
(2)
Where, S is shear stress (MPa), Te is the equivalent torque (Nm), and D is the shaft diameter (mm).
Stress approach of winch motor shaft
The stem-cutting conveyor was folded vertically onto the winch frame in its typical configuration. A 0.12 kW DC winch motor (H9D12-120D, Korea Hoist Co., Ltd., Ansan, Korea) was used to drive the stem-cutting conveyor into a horizontal position while the tractor-mounted radish collector was in use. The winch motor's shaft torque was calculated using equation (3) (Matejic et al., 2013). The equivalent torque under the folded or unfolded stem-cutting conveyor was used to calculate the shear stress and lifespan of the winch motor shaft, which was the same method used for the conveyor motors' shafts.
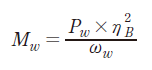 (3)
(3)
Where, Mw is the torque of the winch motor shaft (Nm), Pw is the calculated hydraulic power of the winch motor (0.3, 0.7, 1.1 and 1.4 kW), w is the radial velocity of the winch motor (0.9, 1.4, 2, and 2.2 rad·s-1), ηB is the efficiency ratio of the drum bearings (1), and Dw is the shaft diameter of the winch motor (16 mm).
Analysis of stress behavior of transportation joint
Welding was used to attach the transportation joint of the transfer conveyor (Fig. 1), as dynamic components of equipment can result in serious injuries and fatalities when subjected to repetitive load and oscillation. Tensile strength was tested on the transportation conveyor belt. Torsional stiffness is greatly increased in beams with non-circular cross sections when the end portions are wrapped with stiff blocks (Wikipedia, 2021). The deflection and torsion constant of joint elements were calculated from the measured torque results using equations (4) and (5) under loaded and unloaded conditions.
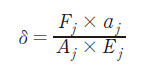 (4)
(4)
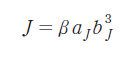 (5)
(5)
Where, δ is the deflection (mm), Fj is the applied force (294.3 N), aj> is the long length of the joint component (m), aj> is the area of the joint component (m2), Ej is the elastic modulus of the joint (MPa), J is the torsional constant, bj is the short length of the joint component (m), β is the numerical factor depending on the ratio of aj>/bj (0.299), Te is the equivalent torque (Nm), and Sj is the maximum shear stress of the joint (MPa).
The Basquin equation was utilized in accordance with the ASTM standard for S275 mild steel to assess stress versus cycle-to-failure data for the transportation joint material (Piotr, 2011). Yield strength and modulus of elasticity were 0.275 and 40 GPa, respectively, for the S275 mild steel. To determine the damage, we used equations (6) and (7) to adjust the material's shear stress from the corresponding torque with load spectrum.
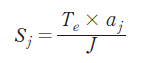 (6)
(6)
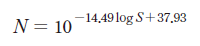 (7)
(7)
Where, Sj is the maximum shear stress of the joint (MPa), Te is the equivalent torque (Nm), J is the torsional constant, aj is the long length of the joint component (m), Nis the number of cycles, and S is shear stress (MPa).
Analysis of stress nature of stem-cutting blade
At the end of the stem-cutting conveyor, a blade for cutting radish stems was attached. When the radish was put onto the conveyor, the stem was clipped using a circular spinning blade operated by a hydraulic motor. Tangential compressive stress and deformation are created at the edge of the circular blade as a result of the central force created by the blade's operating speed. When the stem cutting force is delivered to the cutting blade, the circular blade's tangential stress and deformation can be calculated using equations (8 - 12) through the linear fitting approach (Li et al., 2015).
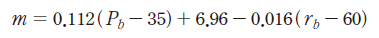 (8)
(8)
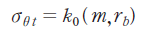 (9)
(9)
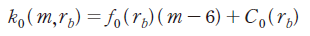 (10)
(10)
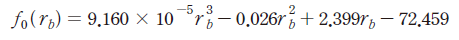 (11)
(11)
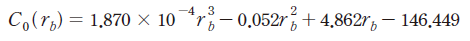 (12)
(12)
Where, is the radial compressive stress (MPa), k0 is the coefficient of function (m, rb), Pb is the blade-driving force (8, 16, 24, 32, 40, and 48 N), m is the deformation (mm), rb is the radius of the stem-cutting blade (0.08 m), and f0 and C0 are the coefficients of function (rb).
The relationship between stress and strain was expressed by equation (13) using power law hardening proposed by Hollomon (Hollomon, 1945). The process of fatigue life with amplitude of elastic strain was described using equation (14) on Ti alloy IMI 834 material (Singh and Singh, 2002).
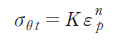 (13)
(13)
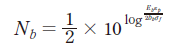 (14)
(14)
Where, K is the strength coefficient of the material (1310.5), ɛp is the amplitude of elastic strain, n is the hardening exponent of strain (0.0463), Nb is fatigue life cycles of the cutting blade, Eb is the blade modulus of elasticity (200 GPa), bb is the burgers vector (0.036), and σf is the fatigue strength coefficient (1,200).
Severity calculation of damage for the collector
The conveyor's workload was investigated using the motor shaft's load signal as torque, stress amplitude, and damage ratios associated with the load spectrum of loaded and unloaded conditions. By subtracting the influence of mean torque, the Smith-Watson-Topper mean stress equation and Rain-flow cycle counting technique were used to determine the appropriate shear stress with distribution of fatigue life cycles and magnitude of load spectrum (Aldeeb and Abduelmula, 2018). Torque magnitude and the total number of fatigue life cycles of the collector’s whole life were computed using equations (15) and (16).
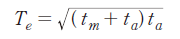 (15)
(15)
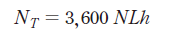 (16)
(16)
Where, Te is the effective torque (Nm), tm is the average torque (Nm), ta is torque amplitude of measurement (Nm), NT is the total number of fatigue cycles, N is the number of computed cycles for the load measurement (cycle·s-1), L is the whole life of the collector (year), and h is the annual utilization hour (h·year-1).
The collector's whole life was considered ten years and its working hours was considered 3,000 hours. The severity level of the process was expressed using equation (17) across the ratio of the sum of damage fractions (Bannanttne et al., 1990). The damage sum was determined using measured load and S-N (bending stress vs. the number of cycles) curve to predict the number of cycles of loading to failure (Fatemi and Yang, 1998). The S-N curve was transformed to a torque-cycle curve due to the damage caused by the torque signal (Nguyen et al., 2011). Miner's theory (Miner, 1945) of normalized fatigue referenced the least amplitude of the damage sum at all working velocities.
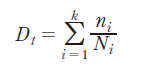 (17)
(17)
Where, Dt is the damaged sum of the operation, ni is the number of actually generated cycles at the ith operation condition, and Ni is the theoretical fatigue life cycles at the ith operation condition.
Selected design variables of the collector
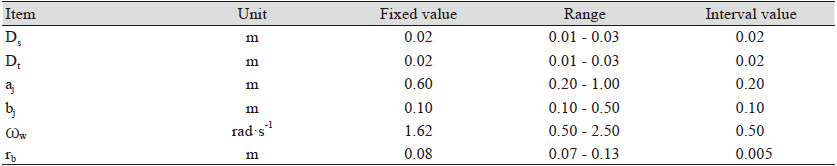
For the manufacturer, an efficient design process and effective material handling are essential to create more efficient and cost-effective machinery that may increase agricultural productivity. The design parameters of the collector’s model, such as shaft diameters of the stem-cutting conveyor motor (Ds), transferring conveyor (Dt), long length (aj), and short length (bj) of the transportation joint, radial speed of the winch conveyor (w), and radius of the stem-cutting blade (rb) were mentioned in Table 1. Various results for shear stress, fatigue life, and deflection of the components was focused under the loaded condition.
Results and Discussion
Stress behavior of conveyor motor shafts
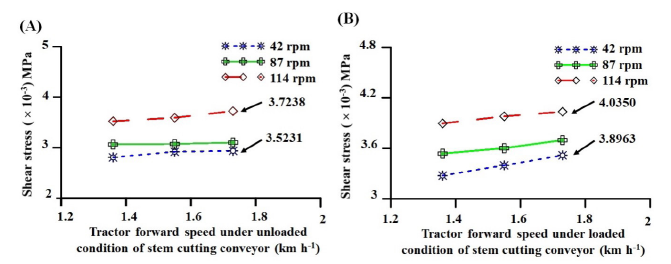
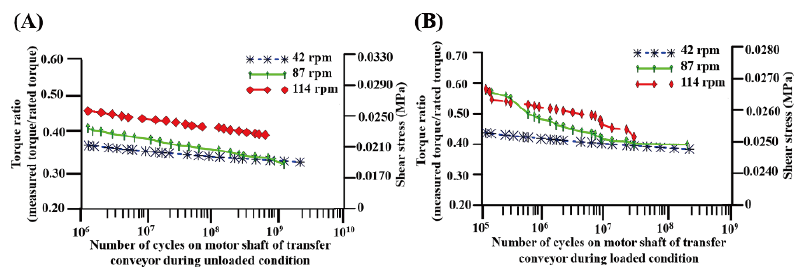
The lowest and highest torque magnitude of the motor shafts for stem-cutting and transferring conveyors under the rotational speed of 114 rpm with a tractor forward speed of 1.73 km·h-1 were 15.4907 to 19.0922 Nm, 18.2304 to 21.2136 Nm, 13.5853 to 15.9828 Nm, and 16.9853 to 19.9827 Nm, respectively, with and without load conditions. Figs 3 and 4 show the shear stress of motor shafts for stem-cutting and transferring conveyors with and without load conditions. With the same rotational and tractor ground speeds, the smallest and largest shear stress of motor shafts were observed as 3.5231 to 3.7238 × 10-3 MPa, 3.8963 to 4.0350 × 10-3 MPa, 2.7713 to 3.0206 × 10-3 MPa, and 3.3525 to 3.5419 × 10-3 MPa, respectively, with and without load conditions.
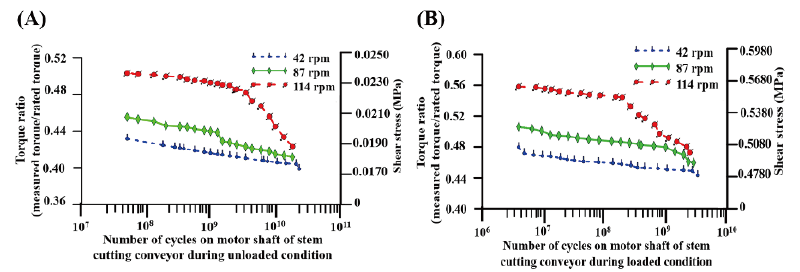
The temporal distribution of the stress spectrum and the load history of the motor shafts of stem-cutting and transferring conveyors are shown in Figs 5 and 6 for all operating and tractor speeds with and without load conditions. The motor shafts were capable of establishing over 107 cycles under all operating circumstances. For each type of equipment, a fatigue limit of maximum load in ten million cycles is permissible (Miner, 1945).
With and without load conditions, the high cycles to low speed and low cycles to high speed were observed. The largest stress distributions of motor shafts at 114 rpm were 0.0235 to 0.0261 MPa and 0.0206 to 0.0242 MPa, respectively. A stepped cylindrical geometry, loading, stress, and deflection, or material strength and stiffness, are taken into consideration while designing a shaft (Kolgiri et al., 2013). The maximum torque ratios of the motor shafts under the rotational speeds of 42 and 114 rpm were obtained as 0.4318 to 0.5017, 0.4797 to 0.5583, 0.4318 to 0.5017, and 0.4367 to 0.5583, respectively, with and without load conditions.
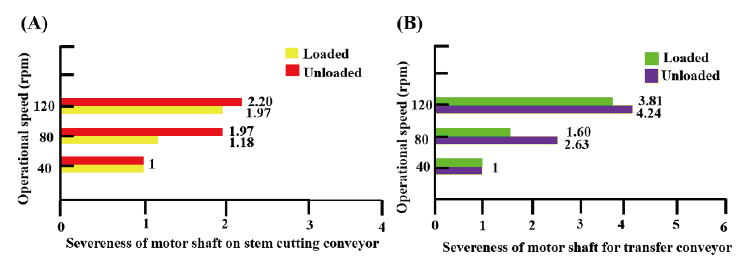
The corresponding severity of the rotational speeds of two motor shafts is displayed in Fig. 7. The severity levels of shafts for stem-cutting and transfer conveyor belts were 1, 1.97, and 2.20; 1, 1.18, and 1.97; 1, 2.63, and 4.24, and 1, 1.60, and 3.81, respectively, with and without load conditions. When the operational speed was increased, the severity level of the damage sum also increased up to 100 - 424% under different speed and load conditions.
Stress behavior of winch motor shaft
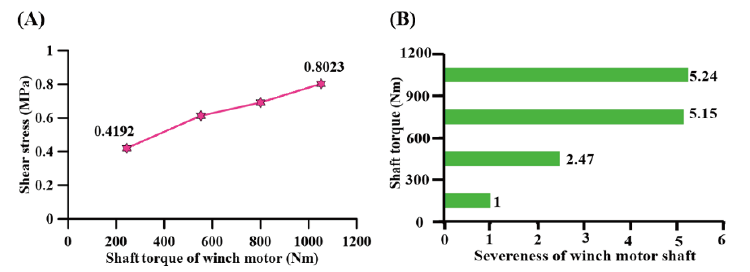
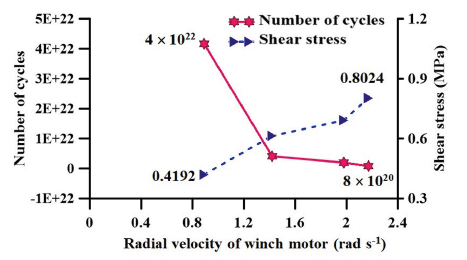
Fig. 8A shows the relationship between shaft torque and shear stress of the winch motor, and Fig. 8B shows the relationship between shaft torque and damage severity of the winch motor. The lowest and highest shear stress of the winch motor shaft were 0.4192 to 0.8023 MPa at the lowest and highest torque positions under loaded conditions. Damage severity levels of the shaft were attained at 1, 2.47, 5.15, and 5.24 related to the shaft torque of 244, 552, 800, and 1,052 Nm, respectively.
Stress behavior of transportation joint
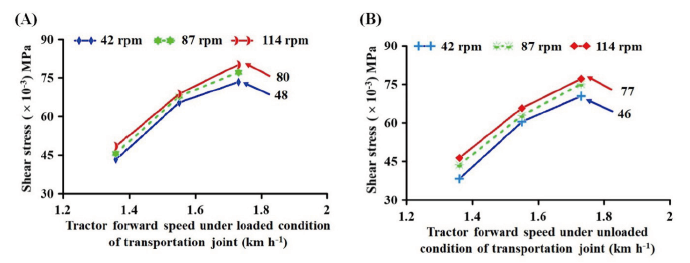
The shear stress of the transportation joint is shown in Fig. 9. The maximum shear stress was found to be 46 × 10-3 to 77 × 10-3 MPa and 48 × 10-3 to 80 × 10-3 MPa at the rotational speed of 114 rpm and tractor speed of 1.73 km·h-1, with and without load conditions. The impact of uncertain alignments on the fatigue strength of 3 to 4 mm deep butt-welded joints was conducted by using mini-scale samples (Fricke et al., 2015) and panels (Lillemäe et al., 2013). For thin-walled building parts, the welding twist can cause considerable high-to-secondary stresses under external loads (Radaj et al., 2006).
The torque and stress amplitude relationship of a transportation joint was investigated with and without load conditions. The observation revealed that under all working conditions, over 107 fatigue life cycles would be generated. The smallest and largest cycles of the joint at 114 and 42 rpm were found to be proportional to the maximum and minimum torque load ratios. At 114 rpm, the maximum amplitudes of shear stresses were 0.0015 and 0.0844 MPa, respectively. At 42, 87, and 114 rpm, the highest ratios of measured torque to rated torque were around 0.4569, 0.4619, and 0.4805, and 0.4809, 0.4864, and 0.4883.
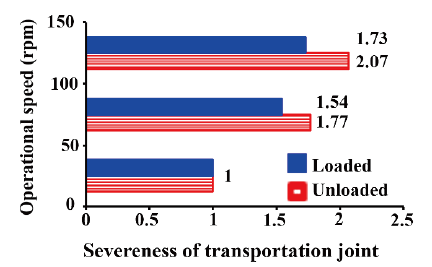
Various severity levels for the transportation joint were approximately 1, 1.77, and 2.07, and 1, 1.54, and 1.73, respectively, with and without load conditions under the rotational velocities of 42, 87, and 114 rpm. Furthermore, the severity level of the joint corresponds to the rotational velocity, as shown in Fig. 10. When the operating speed was higher, the damage level of the joint was increased by about 100 - 207%, with and without load conditions.
Stress behavior of stem-cutting blade
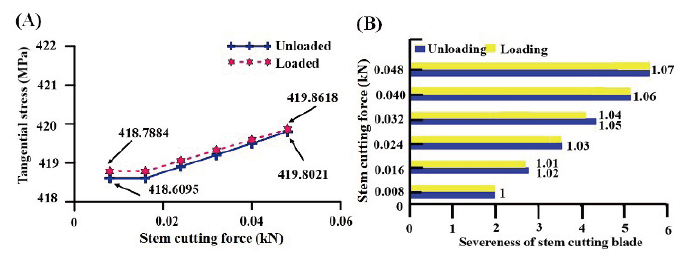
The tangential stress on the stem-cutting blade edge is shown in Fig. 11A. There was a slight increase from 418.6095 to 419.8021 MPa and 418.7884 to 419.8618 MPa by various stem-cutting forces at 8, 16, 24, 32, 40, and 48 N, with and without load conditions. Significant tangential compressive stress at the blade's end results in bucking deflection, which reduces the trimming quality, increases slit waste, and shortens blade life (Li et al., 2015). Fig. 11B shows the damage severity levels of the blade as 1, 1.02, 1.03, 1.05, 1.06, and 1.07, and 1, 1.01, 1.03, 1.04, 1.06, and 1.07, with and without load conditions, respectively.
Effects of design variables of the collector
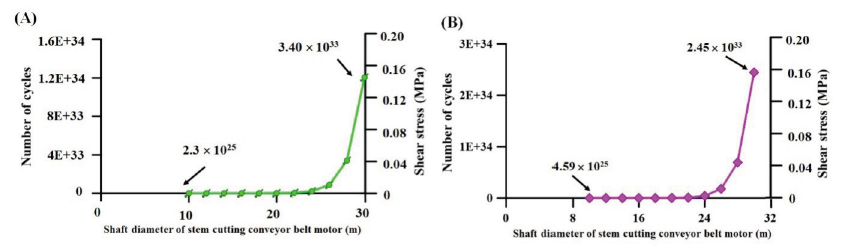
Fig. 12 shows that when the belt motor shaft diameters for stem-cutting and transferring conveyors increased, the shear stress decreased and fatigue life increased. The shear stress decreased continuously between 0.1493 to 0.0055 MPa and 0.1329 to 0.0049 MPa, respectively, and the fatigue life increased up to 3.40 × 1033 and 2.45 × 1033, respectively. Increasing the winch motor's radial velocity led to an increase of the shear stress on the shaft, however, the number of fatigue cycles decreased, as illustrated in Fig. 13. The shear stress on the winch motor shaft increased from 0.4192 to 0.8024 MPa, while the number of fatigue cycles decreased from 4 × 1024 to 8 × 1019. Belt width and pulley diameter can be selected based on the accurate diameter of the shaft.
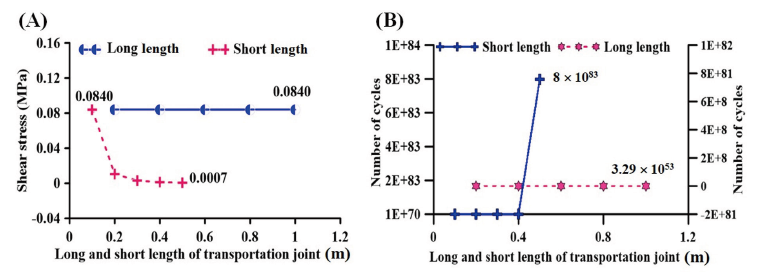
Considering the design variables of the machine, the optimum design structure can be determined from the fatigue strength of the S-N curve for the transportation joint. The shear stress distribution of the long length side was stable at 0.0840 MPa, but the shear stress distribution of the short length side gradually decreased from 0.0840 to 0.0007 MPa, until it became ignored (Fig. 14A). However, the fatigue life cycles of the transportation joint were fixed at 3.29 × 1053 for both the short and long length sides, but in the case of the short length side, it continuously increased to 8 × 1083 (Fig. 14B). During the stress condition, the deflection of the transportation joint sharply decreased to between 221 × 10-6 to 44 × 10-6 mm and 74 × 10-6 to 15 × 10-6 mm for the long and short length sides, respectively. According to the Korean steel structural design code and commentary (Korean Society of Steel Structure, 2018), a distortion of one-third of the length is considered acceptable. As such, the complete deflection was not obvious.
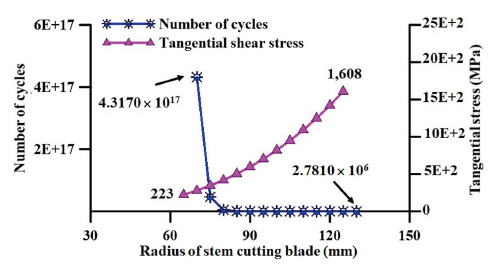
In addition, the tangential stress on the stem-cutting blade was between 223 and 1,608 MPa, but the number of cycles decreased from 4.3 × 1017 to 2.78 × 106 with the increase of the blade radius as shown in Fig. 15. Deflection of the blade varied from 2.88 to1.92 mm, which was insignificant. The tangential shear stress was surveyed using an X-ray stress meter for three different types of circular saw blades (Umetsu et al., 1994).
The experimental findings demonstrated that even when the tension between the components is negligible, a fatigue study is necessary (1 MPa or less). However, repetitive low-level loading may develop and expand a fatigue fracture at a stress concentration, eventually resulting in failure or even deformation. Fatigue fractures usually begin at stressors, and in the case of agricultural machinery, even a small amount of fatigue may result in significant damage later on. As a result, eliminating such faults boosts fatigue strength.
Conclusions
In this study, the stress and fatigue of the main components of the radish collector were investigated under dynamic load conditions in order to predict the expected machine life, design, and size of the materials used in the collector. Considering the field condition, an experimental study was conducted to determine the shaft torque with and without load conditions. The Smith-Watson-Topper mean stress equation and the rain-flow-counting technique were both utilized to determine the necessary shear stress in the presence of a distribution of fatigue life cycles. The severity of the procedure was determined using Miner's rule.
Under running conditions, shear stress was found to be insignificant for the motor shafts of the stem-cutting conveyor belt, transfer conveyor belt and winch, transportation joint, and stem-cutting blade. All running conditions could produce more than 107 high cycle fatigue strengths. Furthermore, the highest severity levels of motor shafts for stem-cutting and transferring, transportation joint, and cutting blades were 2.20, 4.24, 2.07, and 1.07, and 1.97, 3.81, 1.73, and 1.07 with and without load conditions, respectively, aside from 5.24 for the winch motor shaft under loaded conditions.
Regarding the design parameters, for stem-cutting and transfer conveyors, increasing the diameter of the belt motor shaft resulted in lower shear stress and longer fatigue life. Although shear stress on the shaft increased, fatigue cycles were reduced when the winch motor's radial velocity was increased. The shear stress and fatigue life cycles of the transportation joint were steady across the long length side, demonstrating that this prototype is capable of working safely in the field.
The experimental findings of this study provide valuable information for the development of an automated conveyor system for radish collectors. For example, the findings may be applied to the design of appropriate radish collection conveyor components, resulting in increased design dependability while simultaneously lowering material costs.
Acknowledgments
This work was supported by the Korea Institute of Planning and Evaluation for Technology in Food, Agriculture, and Forestry (IPET) through the Agriculture, Food and Rural Affairs Convergence Technologies Program for Educating Creative Global Leaders, funded by the Ministry of Agriculture, Food and Rural Affairs (MAFRA) (Project No. 320001-4), Republic of Korea.
Authors Information
Khine Myat Swe, https://orcid.org/0000-0002-6565-4932
Md Nasim Reza, https://orcid.org/0000-0002-7793-400X
Milon Chowdhury, https://orcid.org/0000-0002-9887-7980
Mohammod Ali, https://orcid.org/0000-0002-1822-3005
Sumaiya Islam, https://orcid.org/0000-0003-3180-5699
Sang-Hee Lee, https://orcid.org/0000-0003-2028-9035
Sun-Ok Chung, https://orcid.org/0000-0001-7629-7224
Soon Jung Hong, https://orcid.org/0000-0003-2584-5379